Cooler Master Seidon 120 XL Watercooler Review

The Cooler Master Seidon 120 XL is designed for all Intel Socket LGA2011 / 1366 / 1156 / 1155 / 775 and Athlon 64 processors. Here is an overview of the system and testing methodology.

Asus P9X79 - X79 Chipset
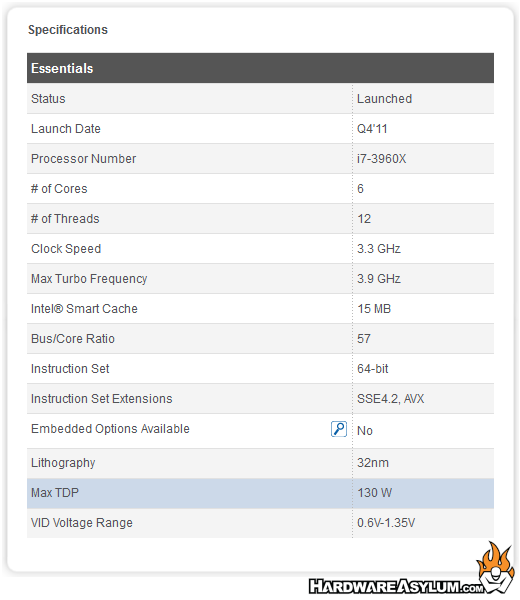
Intel Core i7 3960x (3.3Ghz) Hex Core 6 x 256KB L2 Cache 15MB L3 Cache
Cooler Master Seidon 120 XL
Thermaltake Water 2.0 Pro

The CPUID System Monitor was used to obtain and record system temperature data and being that this is a quad core processor we need something that will work across all of the cores at once.  For this task we're using a new version of Prime95 (p95v255a) that will allow you to spawn (n) instances to test with.

Editors note: Even though the Windows 7 task manager reported 100% processor usage we could never attain a 100% of the rated heat output as documented by Intel (see below) when using Prime95 as a basis for that heat production. Knowing this we ran the stress test until the maximum temperature was attainted and stabilized.

Other things to consider when judging software induced heat output.
a) Clock throttling by the processor at high temperatures.
b) Normal software isn't designed to produce maximum heat output.
c) Variances of cooling temperature.
d) Variances in CPU load.
e) Inaccuracies in thermal diode readouts.
Of course the list goes on..

Our testing methodology is aimed to provide a real world look into this heatsink given the test system provided.

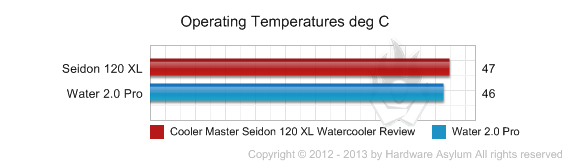
A C/W rating can quickly be calculated using this formula.

C/W = (CPU temp - Ambient temp)/(Variance(%) * CPU Watts)
Allowed variance for this test = 85%
CPU Watts = 130W

0.20C/W = (47C - 25C)/(.85(130W))

For this next test the CPU speed was cranked up to 4.6Ghz and the test was re-run.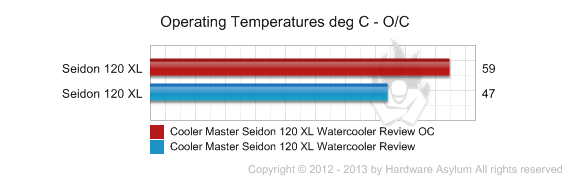

To calculate a new C/W rating for this test we will need to factor in the increased processor wattage. The formula and constants for this are listed below.

ocC/W = dCPU Watts * (ocMhz / dMhz) * (ocVcore / dVcore)2
ocMhz = 4600
dMhz = 3300
ocVcore = 1.375
dVcore = 1.2
The variance still applies for our C/W calculation
Allowed variance for this test = 85%
CPU Watts = 238W

0.17 C/W = (59C - 25C)/(.85(238W))

In our heatsink and waterblock tests we don't really focus on overall load temperatures but rather how well the product can remove heat given a specified heat load. Since this is a real world testing method we need to take into consideration real world variables and estimate tolerances. This is why we normally only apply 85% of the total wattage output to our heat calculations.

The resulting C/W number is used to rate how efficient a heatsink or waterblock is based on the given heat load. These numbers can be used to determine heat capacity, the larger the difference the less efficient the heatsink is. (aka not good for overclocking)

Here we have a watercooling system that can clearly handle more than our system can dish out.  All too often we find a rather pronounced variance between the default and overclocked speeds which can sometimes be good and sometimes be bad.  For these tests you'll see that the resulting C/W numbers went down between our tests which is a good thing indicating that we haven't reached the thermal saturation point.  

It should be noted that some of the above performance is due in part to the Asus turbo fan profile which will control the fan so that you get a low noise under normal use and more fan performance when things heat up.  Under our OC tests the fans were spinning in excess of 2000rpm which is a testament to how much heat this system was producing and the power of the Cooler Master fans.    

Keep in mind these calculations are provided for demonstration purposes only and may not reflect the actual lab tested C/W rating, but we're pretty close.